Math Questions 15.7 - Transforming Graphs
The following math revision questions are provided in support of the math tutorial on Transforming Graphs. In addition to this tutorial, we also provide revision notes, a video tutorial, revision questions on this page (which allow you to check your understanding of the topic and calculators which provide full, step by step calculations for each of the formula in the Transforming Graphs tutorials. The Transforming Graphs calculators are particularly useful for ensuring your step-by-step calculations are correct as well as ensuring your final result is accurate.
Not sure on some or part of the Transforming Graphs questions? Review the tutorials and learning material for Transforming Graphs
| Tutorial ID | Title | Tutorial | Video Tutorial | Revision Notes | Revision Questions |
|---|---|---|---|---|---|
| 15.7 | Transforming Graphs |
Transforming Graphs Revision Questions
1. . What is the parent function of
- x/1 - x
- 1/1 - x
- 1/x
- 1 + 1/1 - x
Correct Answer: C
2. . The graph of the function y = x2 + 4x + 5 is obtained by translating the y = x2 graph by
- 2 units right and 1 unit up
- 2 units left and 1 unit up
- 2 units down and 1 unit up
- 1 unit right and 2 units up
Correct Answer: B
3. . One of the graph's points of the function y = 2x2 - 1 is at (2, 7). Where is the corresponding point in the graph of the function y = 2x2 - 8x + 7.
Hint! Determine the type of graph's transformation taking place in the second function.
- (2, 9)
- (4, 7)
- (4, 9)
- (0, 0)
Correct Answer: B
4. . What is the formula of the unknown function f(x) in the figure?
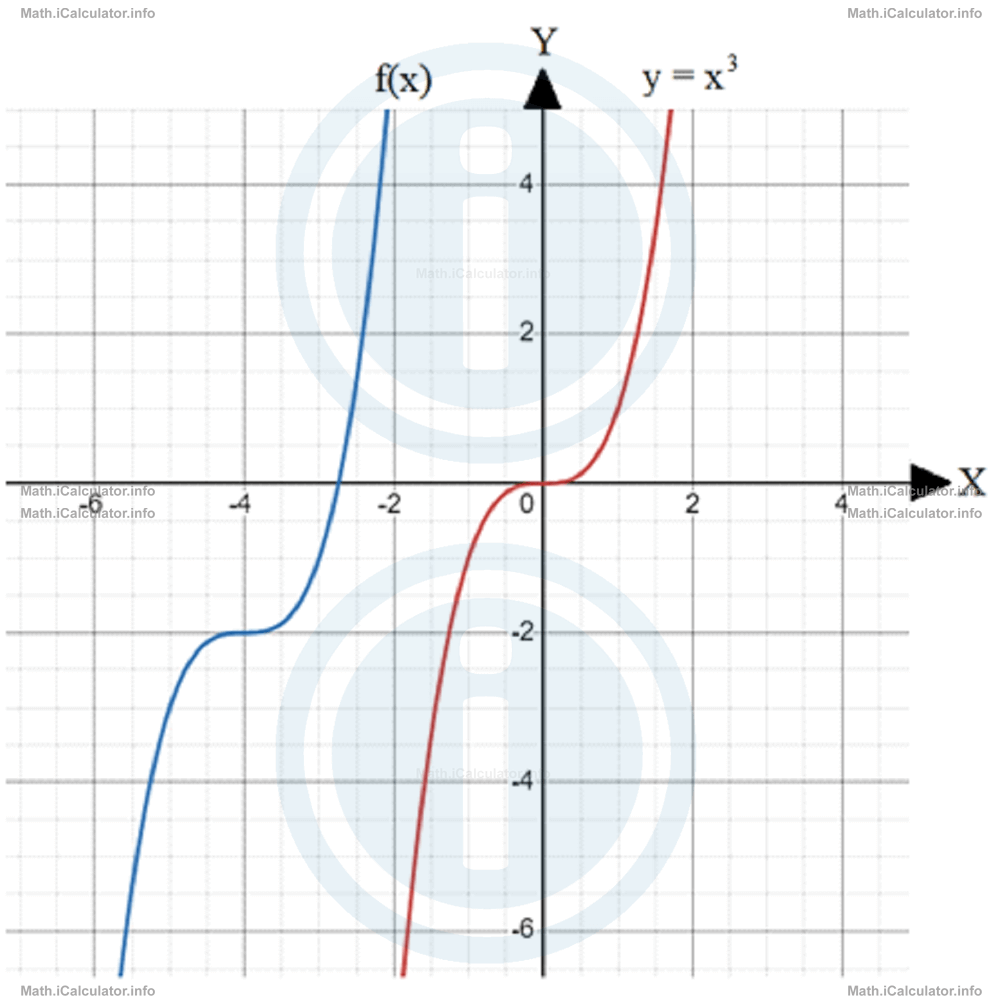
- f(x) = (x - 4)3 + 2
- f(x) = (x + 4)3 + 2
- f(x) = (x + 4)3 - 2
- f(x) = (x + 2)3 - 4
Correct Answer: C
5. . The function y = 4x + 3 is obtained by displacing the function
- y = x + 3 by 4 units upwards
- y = 4x by 3 units on the left
- y = x by 3 units upwards
- y = 4x by 3 units upwards
Correct Answer: D
6. . What is the unknown function f(x) in the figure?
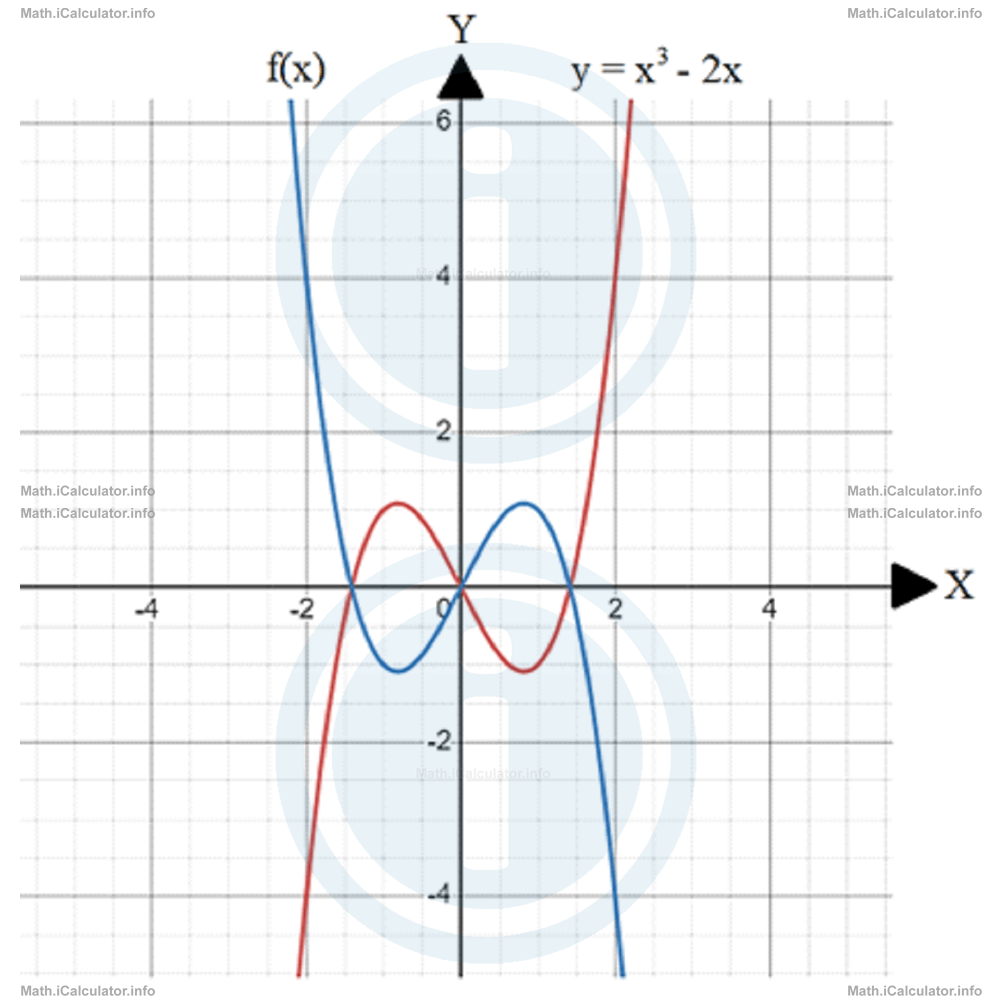
- f(x) = x3 + 2x
- f(x) = -x3 - 2x
- f(x) = -x3 + 2x
- f(x) = x3 - 2x
Correct Answer: C
7. . What is the correct formula for the horizontal reflection of the function
- y = -x3 + 2x + e=x
- y = -x3 + 2x - ex
- y = -x3 + 2x + e=x
- y = x3 - 2x - e=x
Correct Answer: A
8. . The graph of the function y = 5 - 2x + 3x2 is displaced by 3 units due right and 2 units down. What is the formula of the new function obtained?
- y = 3x2 + 20x + 40
- y = 3x2 - 20x + 40
- y = 3x2 + 20x + 38
- y = 3x2 - 20x + 38
Correct Answer: A
9. . What is the formula of the unknown graph f(x) in the figure?
- f(x) = -x2 + 2x - 3
- f(x) = x2 - 2x - 3
- f(x) = -x2 + 2x + 3
- f(x) = -x2 - 2x - 3
Correct Answer: D
10. . What is the formula of the double reflection of the function y = 2x3 + x2 + 5x - 3?
- f(x) = 2x3 - x2 - 5x - 3
- f(x) = 2x3 - x2 + 5x + 3
- (x) = -2x3 - x2 - 5x - 3
- f(x) = 2x3 - x2 + 5x + 3
Correct Answer: B
Whats next?
Enjoy the "Transforming Graphs" practice questions? People who liked the "Transforming Graphs" practice questions found the following resources useful:
- Practice Questions Feedback. Helps other - Leave a rating for this practice questions (see below)
- Types of Graphs Math tutorial: Transforming Graphs. Read the Transforming Graphs math tutorial and build your math knowledge of Types of Graphs
- Types of Graphs Revision Notes: Transforming Graphs. Print the notes so you can revise the key points covered in the math tutorial for Transforming Graphs
- Check your calculations for Types of Graphs questions with our excellent Types of Graphs calculators which contain full equations and calculations clearly displayed line by line. See the Types of Graphs Calculators by iCalculator™ below.
- Continuing learning types of graphs - read our next math tutorial: Gradient of Curves
Help others Learning Math just like you
We hope you found this Math tutorial "Transforming Graphs" useful. If you did it would be great if you could spare the time to rate this math tutorial (simply click on the number of stars that match your assessment of this math learning aide) and/or share on social media, this helps us identify popular tutorials and calculators and expand our free learning resources to support our users around the world have free access to expand their knowledge of math and other disciplines.