Math Lesson 15.3.3 - Finding the Number of Wiggles of a Cubic Function Graph without Plotting It

Please provide a rating, it takes seconds and helps us to keep this resource free for all to use

Welcome to our Math lesson on Finding the Number of Wiggles of a Cubic Function Graph without Plotting It, this is the third lesson of our suite of math lessons covering the topic of Cubic Graphs, you can find links to the other lessons within this tutorial and access additional Math learning resources below this lesson.

Finding the Number of Wiggles of a Cubic Function Graph without Plotting It

We are not always interested in plotting the graph of a cubic function, especially when the question simply requires us to express a rough opinion about the features of the function's graph. In that case, one of the graph features we can determine without needing to plot it consists of the number of wiggles. This number depends on the number of roots the cubic equation has (we have called them the number of zeroes), which corresponds to the number of x-intercepts of the graph.

As always, we consider the simplified version of the original cubic function where y is taken as zero. Then, we follow the procedure described below:

Step 1: Remove the constant d from the original equation. This avoids situations where there are wiggles on the same part of the y-values (for example, on the positive part of the Y-axis only) - a situation that makes us impossible to identify them (the wiggles). In this case, you obtain a similar graph to the original but slightly displaced and stretched/compressed.

Step 2: Find the number of roots of the new equation. If it has a single root, the graph has one wiggle and if it has more than one root, the graph has two wiggles.

For example, the figure below shows two graphs: y = x³ - 3x + 4 and y = x³ - 3x, which you can easily see that are very similar to each other.

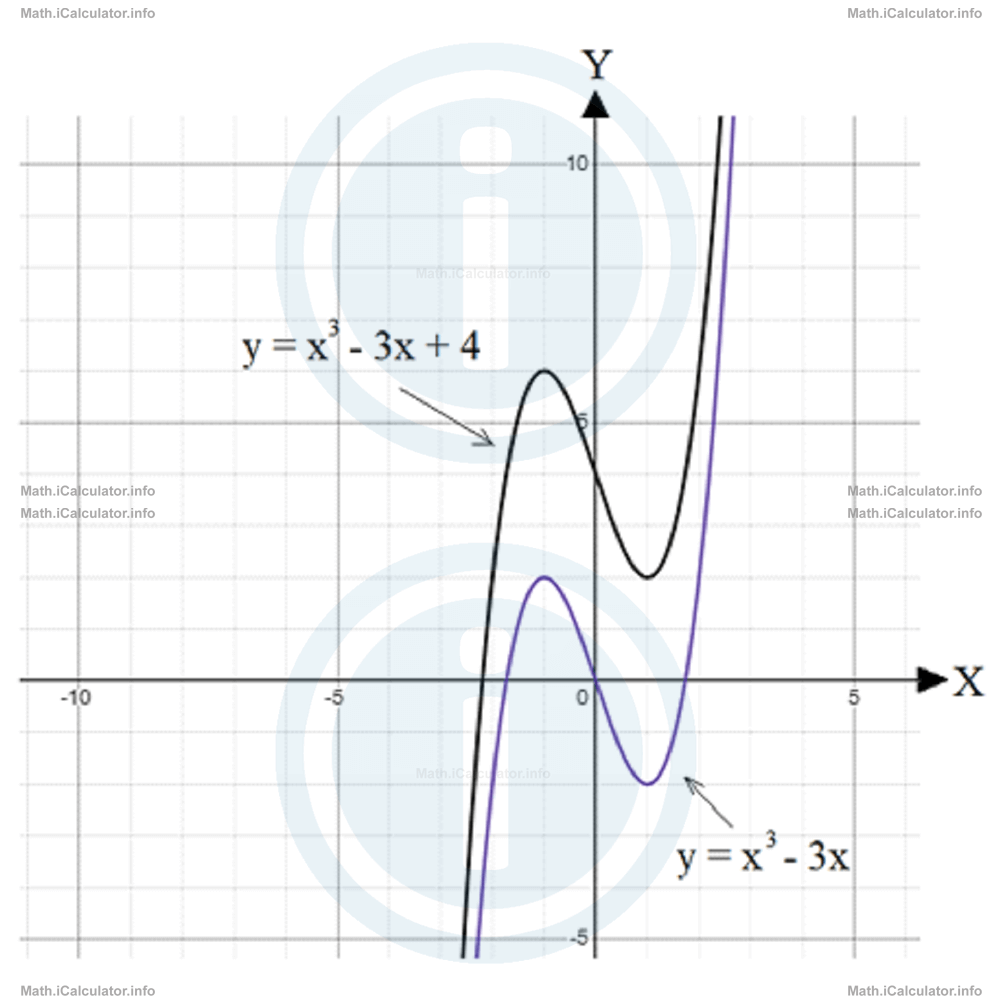
If we solve the one-variable version of the original equation x³ - 3x + 4 = 0, we obtain a single root (from the graph, you can see that it is slightly less than -2). This may lead to the wrong conclusion that the graph has only one wiggle. However, by solving the version of the equation without the constant d = -4, we obtain three roots - a clue which leads to the conclusion that the graph has two wiggles.

Indeed, we have

This equation is true for x = 0 and for x² - 3 = 0, i.e. for x² = 3. This gives

Therefore, the x-intercepts of the new equation are (-√3, 0), (0, 0) and (√3, 0).

Example 4

Without plotting the graph, find the number of wiggles of the line

Solution 4

Removing the constant c yields

The corresponding one-variable equation is

It has a single root (x = 0) because the expression in brackets cannot become zero for any value of x. This is because

which is impossible to calculate in the set of the real numbers, as no number raised in square gives a negative result.

Now let's recap everything explained in this tutorial through another example.

Example 5

Write all features of the graph produced by the cubic equation with two variables

including:

1. Direction of the line
2. Root(s)
3. x-intercept(s)
4. y-intercept
5. Number of wiggles

Solution 5

1. From the equation, we identify the following values: a = 1, b = -3, c = 2 and d = 4. Since the coefficient a is positive, this means the graph comes from minus infinity due bottom-left and extends to plus infinity due top-right.
2. With the actual knowledge, we don't have other options to find the root(s) but the iterative methods. (We will explain in the future chapters how to find everything we need using derivatives). Thus, we have to check for any change in the sign of the equation in given intervals. For example, we can make a table with x-values ranging from -5 to + 5 with an increment of 1 unit. In this way, we find the following y-values by substituting each x-value in the original equation: 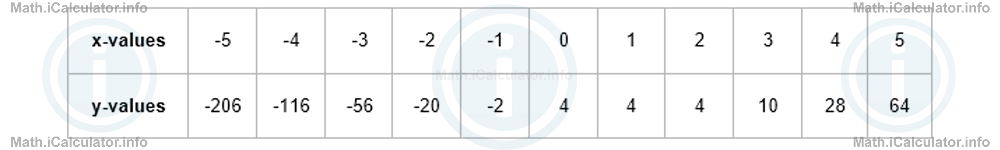From the table, we can identify a single change in sign between -1 and 0. Therefore, the equation has a single root, which we must seek in this interval. We will stop rounding the result to one decimal place. Thus, using the half-interval method, we find for x = -0.5:
   y(-0.5) = (-0.5)³ - 3 ∙ (-0.5)² + 2 ∙ (-0.5) + 4
   = 2.125
   Thus, the change in sign is between -1 and -0.5. Again, for x = -0.6, we have
   y(-0.6) = (-0.6)³ - 3 ∙ (-0.6)² + 2 ∙ (-0.6) + 4
   = 1.504
   For x = -0.7, we have
   y(-0.7) = (-0.7)³ - 3 ∙ (-0.7)² + 2 ∙ (-0.7) + 4
   = 0.787
   For x = -0.8, we have
   y(-0.8) = (-0.8)³ - 3 ∙ (-0.8)² + 2 ∙ (-0.8) + 4
   = -0.032
   Hence, since here we have a change in sign and the result of y(-0.8) is much closer to zero than for y(-0.7), we take the value x = -0.8 as the only root for the original equation.
3. From theory, it is known that any root of the cubic equation with one variable
   ax³ + bx² + cx + d = 0
   is an x-intercept for the corresponding cubic equation with two variables
   y = ax³ + bx² + cx + d
   Therefore, we have only the point A(-0.8, 0) as an x-intercept.
4. The y-intercept is at x = 0. From theory, it is known that this value corresponds to the constant d. Thus, we have y_int = d = 4. Therefore, the graph intercepts the Y-axis at B(0, 4).
5. To calculate the number of the graph wiggles, we remove the constant d = 4 from the original equation and solve for y = 0 the new equation obtained. Thus, since the original equation is
   y = x³ - 3x² + 2x + 4
   we obtain after the above transformations
   x³ - 3x² + 2x = 0
   x(x² - 3x + 2) = 0
   This equation is true for x = 0 and for x² - 3x + 2 = 0. Solving this quadratic equation yields
   x² - 3x + 2 = 0
   x² - 2x + 1-x + 1 = 0
   (x² - 2x + 1) - (x - 1) = 0
   (x- 1)² - (x - 1) = 0
   (x - 1)(x - 1 - 1) = 0
   (x - 1)(x - 2) = 0
   Therefore x = 1 and x = 2 are two other roots besides x = 0 for the "modified" equation above. This means this equation has three roots. Consequently, the graph of the original equation has two wiggles, as explained in theory. Look at the graph of the original equation plotted through a graphing software.

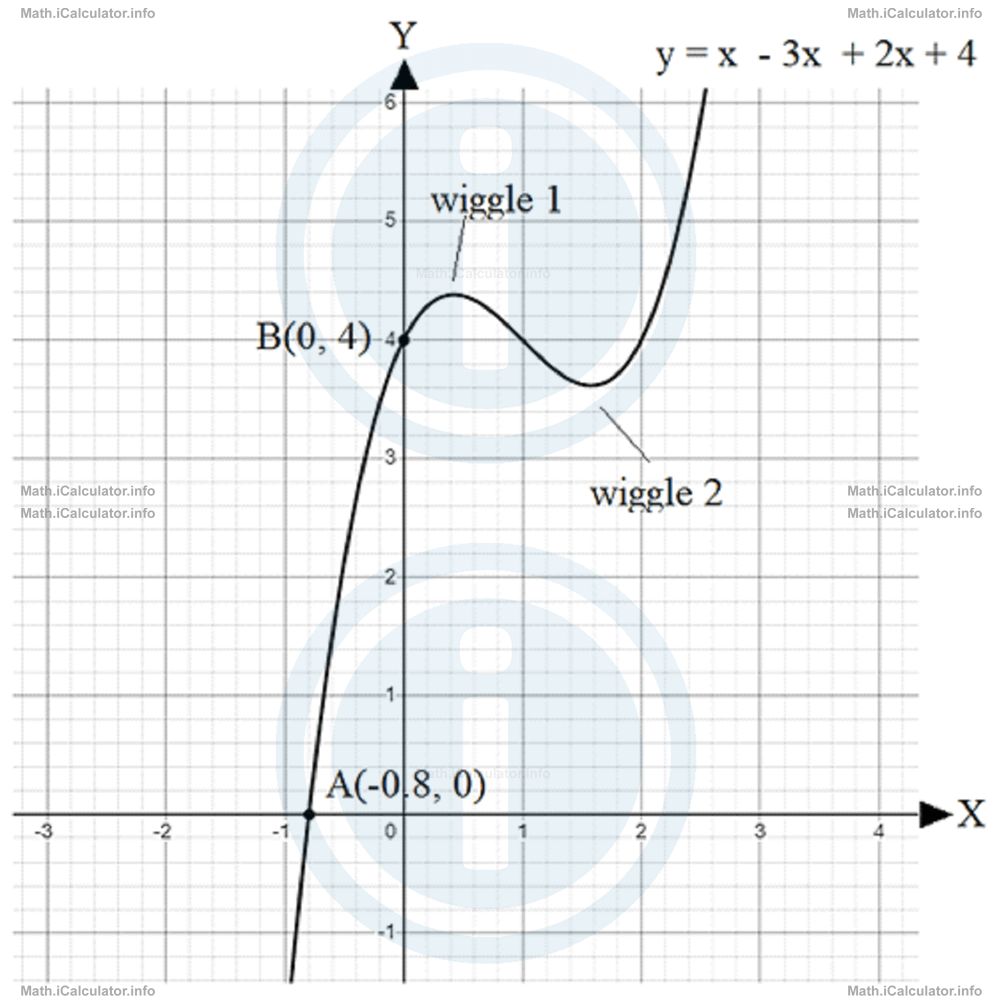
Remark! Not all the information related to cubic function is provided in this tutorial. Consider it as an introduction to cubic graphs, as you will learn many more things about them in this chapter and in other chapters of this course. For example, we will explain how to calculate the coordinates of the local minimum/maximum, how to find the relevant points needed to plot a cubic graph, how to calculate the gradient of a cubic graph at any point, how to plot a more complex cubic graph taking the basic cubic function as a reference, etc.

You have reached the end of Math lesson 15.3.3 Finding the Number of Wiggles of a Cubic Function Graph without Plotting It. There are 3 lessons in this physics tutorial covering Cubic Graphs, you can access all the lessons from this tutorial below.

More Cubic Graphs Lessons and Learning Resources

Whats next?

Enjoy the "Finding the Number of Wiggles of a Cubic Function Graph without Plotting It" math lesson? People who liked the "Cubic Graphs lesson found the following resources useful:

1. Wiggles Feedback. Helps other - Leave a rating for this wiggles (see below)
2. Types of Graphs Math tutorial: Cubic Graphs. Read the Cubic Graphs math tutorial and build your math knowledge of Types of Graphs
3. Types of Graphs Revision Notes: Cubic Graphs. Print the notes so you can revise the key points covered in the math tutorial for Cubic Graphs
4. Types of Graphs Practice Questions: Cubic Graphs. Test and improve your knowledge of Cubic Graphs with example questins and answers
5. Check your calculations for Types of Graphs questions with our excellent Types of Graphs calculators which contain full equations and calculations clearly displayed line by line. See the Types of Graphs Calculators by iCalculator™ below.
6. Continuing learning types of graphs - read our next math tutorial: Reciprocal Graphs

Help others Learning Math just like you

Please provide a rating, it takes seconds and helps us to keep this resource free for all to use

We hope you found this Math tutorial "Cubic Graphs" useful. If you did it would be great if you could spare the time to rate this math tutorial (simply click on the number of stars that match your assessment of this math learning aide) and/or share on social media, this helps us identify popular tutorials and calculators and expand our free learning resources to support our users around the world have free access to expand their knowledge of math and other disciplines.

Types of Graphs Calculators by iCalculator™